Mo logo [home] [lexicon] [problems] [tests] [courses] [auxiliaries] [notes] [staff] german flag
Mathematik-Online lexicon:

Influence of Ordering Variables within Integration

A B C D E F G H I J K L M N O P Q R S T U V W X Y Z overview
The function

$\displaystyle f(x,y)=y \cos \left(x^2\right) $

will be integrated over the region

$\displaystyle V:\quad 0\le x\le 1\,,\quad 0 \le y \le \sqrt{x} \, . $

\includegraphics[width=0.4\moimagesize]{bsp_pol_raender1}

By Fubini's theorem the integral can be calculated by the iterated integral:

$\displaystyle \int\limits_V f$ $\displaystyle =$ $\displaystyle \int\limits_{0}^1\left(\int\limits_{0}^{\sqrt{x}} y \cos \left(x^... ...^1 \left[ \frac{y^2}{2} \cos \left( x^2 \right) \right]_{y=0}^{y=\sqrt{x}}\, dx$  
  $\displaystyle =$ $\displaystyle \int\limits_0^1 \frac{1}{2} x \cos \left( x^2 \right) \, dx = \left[ \frac{1}{4} \sin \left( x^2 \right) \right]_0^1 = \frac{1}{4} \sin 1\,.$  

Changing the order of integration and adapting the integration limits the integral may be also written as

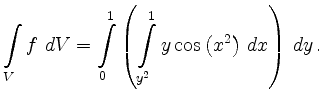
$\displaystyle \int\limits_V f \ dV = \int\limits_{0}^1\left(\int\limits_{y^2}^{1} y \cos \left(x^2 \right)\, dx \right) \, dy\, . $

But in this case it is not possible to calculate the inner integral explicitly. This shows that the ordering of the integration variables can be essential for its evaluation.

(Authors: Höfert/Kimmerle)

see also:

  automatisch erstellt am 20.  8. 2008