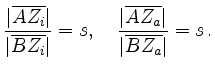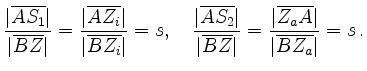The equation
describes a circle in the Gaussian plane.
Its center is
and the radius given by
If 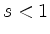 ,
, 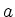 is located
in the interior of the circle,
while
is located
in the interior of the circle,
while 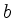 is lies on its outside,
vice versa for
is lies on its outside,
vice versa for 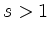 .
.
The circle's parametric representation is given by
Interpreting the equation
geometrically, it asserts that
the distances of a point 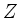 from
two given points
from
two given points 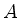 and
and 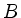 have a fixed ratio
have a fixed ratio  ,
i.e.,
In order to determine the points for which this
relationship holds, we consider, e.g., the case
,
i.e.,
In order to determine the points for which this
relationship holds, we consider, e.g., the case
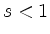 , and use the following auxiliary construction.
, and use the following auxiliary construction.
Referring to the figure, we denote by
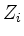 and
and  the points on the line
the points on the line 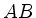 with
with
Moreover, we define two points 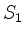 and
and 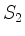 as
the intersections of the line parallel to
as
the intersections of the line parallel to 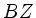 through
through 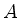 with the line
with the line 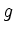 through
through 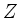 and
and 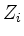 and the line
and the line 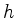 through
through 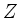 and
and  ,
respectively.
Then it follows from the intercept theorem that
Since
,
respectively.
Then it follows from the intercept theorem that
Since
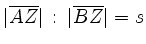 as
well, the line segments
as
well, the line segments
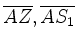 and
and
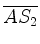 have the same length.
Moreover,
have the same length.
Moreover, 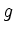 and
and 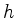 are
orthogonal.
Hence,
are
orthogonal.
Hence,  ,
, 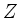 ,
, 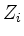 form
a right triangle, and all points
form
a right triangle, and all points 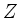 fulfilling these requirements are located
on the circle with
diameter
fulfilling these requirements are located
on the circle with
diameter
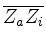 .
.
This geometric argument is due to Apollonius
(200 BC), which is why
such a circle is called an Apollonius circle.
(Authors: Hörner/Abele )
| |
automatisch erstellt
am 11. 6. 2007 |





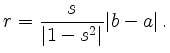
![\includegraphics[width=10cm]{kreis_komplexe_ebene}](/inhalt/aussage/aussage385/img8.png)
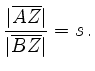
![\includegraphics[width=10cm]{kreis_des_apollonius}](/inhalt/erlaeuterung/erlaeuterung57/img8.png)
![]() and
and ![]() the points on the line
the points on the line ![]() with
with