Mathematik-Online lexicon: Drift of an Airplane

	[home] [lexicon] [problems] [tests] [courses] [auxiliaries] [notes] [staff]
	Mathematik-Online lexicon:
	Drift of an Airplane

A B C D E F G H I J K L M N O P Q R S T U V W X Y Z

overview

An airplane travels with speed km/h due east while the wind velocity is km/h from WSW.

$\includegraphics[width=.8\linewidth]{b_flugzeug_drift}$

In the picture east is the direction of the horizontal axis and north is the direction of the vertical one. The velocity vector of the air plane itself is therefore

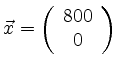
$\displaystyle \vec{x}=\left(\begin{array}{c}800 \\ 0 \end{array}\right)$

and the vector of the wind velocity is given by

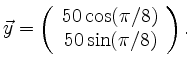
$\displaystyle \vec{y}=\left(\begin{array}{c} 50\cos(\pi/8) \\ 50\sin(\pi/8) \end{array}\right) .$

Starting at the coordinates of the air plane are after one hour (superposition of the motions)

$\displaystyle \vec{x}+\vec{y}=\left(\begin{array}{c} 800+ 50\cos(\pi/8) \\ 50\s... ...ray}\right) \approx \left(\begin{array}{c} 846.19 \\ 19.13 \end{array}\right) .$

If $\vec{p}$ denotes the orthogonal projection of $\vec{y}$ on the line with direction $\vec{x}$ , then the velocity of the air plane relative to the earth is the sum of the component due east

$\displaystyle \vec{x}+\vec{p}=\left(\begin{array}{c} 800+ 50\cos(\pi/8) \\ 0 \end{array}\right) \approx \left(\begin{array}{c} 846.19 \\ 0\end{array}\right)$

and the drift

$\displaystyle \vec{d}=\vec{x}+\vec{y}-(\vec{x}+\vec{p})=\left(\begin{array}{c} ... ...end{array}\right) \approx \left(\begin{array}{c} 0 \\ 19.13 \end{array}\right)$

towards north.

()

[Links]

automatisch erstellt am 28. 3. 2008